The Basics of CT
CT serves as a “connective tissue” between computer science and a variety of other fields (Martin, 2018). CT’s interaction with traditional STEM subjects, for example, has resulted in new multidisciplinary fields like computational geometry and bioinformatics (Lee et al., 2020). CT is a problem-solving and instructional method in which solutions are expressed as algorithms (small computing steps) that a computer can carry out (Aho 2012; Grover and Pea, 2013). CT has been introduced into primary and secondary education around the world during the last decade, and it is being marketed as a necessary skill for every K-12 student (Grover and Pea, 2018).
CT’s Framework
Peel and colleagues presented this CT taxonomy at the CTE-STEM conference, which we found very useful in framing our ideas about CT deployment in Singapore:
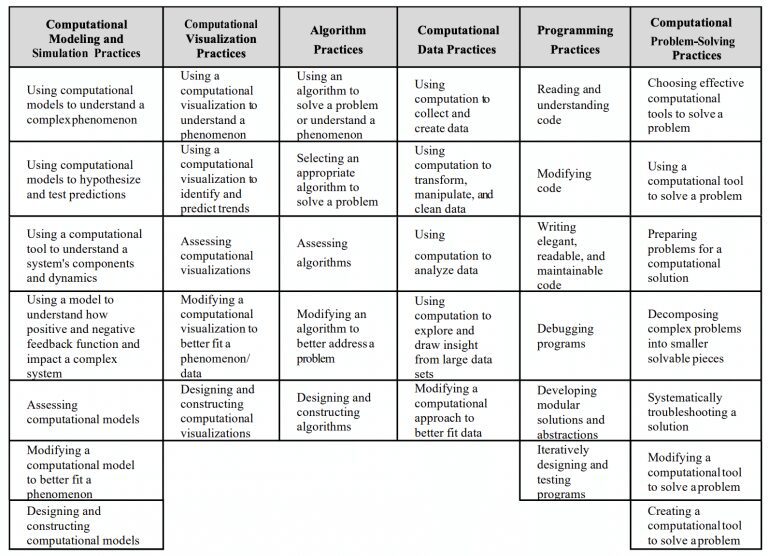
Students can use computational models and simulations to visualize a phenomenon, comprehend the dynamics of a system, and test predictions and hypotheses. Students gain a better knowledge of phenomena by designing and building models (Wilensky & Reisman, 2006). NetLogo and PhET are two pieces of software designed to make mathematics and scientific simulations more approachable.
Visualization is a metacognitive talent in which students evaluate and comprehend data using graphs, tables, diagrams, static models, and other tools (Gilbert, 2005). Students can use visualization to think about a phenomenon in new ways, especially if the visualization offers an alternative perspective on the topic.
Data practices include data collecting, cleansing, transformation, and analysis, with students manipulating their data using pre-programmed algorithms or writing their own code. Programming, which overlaps with data practices, is the act of writing code, which includes designing, debugging, and testing. Students would also be taught programming practices such as the use of comments to annotate code.
Finally, for problem-solving, students must be able to recognize different ways to address an issue computationally and select an appropriate computational tool to solve the problem. Students may need to break down problems into smaller chunks and manipulate raw data in order to execute a problem-solving algorithm.
Singapore’s CT
Beyond the Computing course, Singapore’s Ministry of Education has acknowledged the importance of incorporating CT into the obligatory curriculum, particularly in math classes. CT is defined as follows by the Curriculum Planning and Development Division (CPDD) in Singapore: Computational thinking is defined as the process of formulating issues and finding solutions that can be realized using a computer (Wing, J. M., 2006). In general, CT refers to the ability to solve issues using four skills: abstraction, decomposition, generalization, and algorithmic thinking.
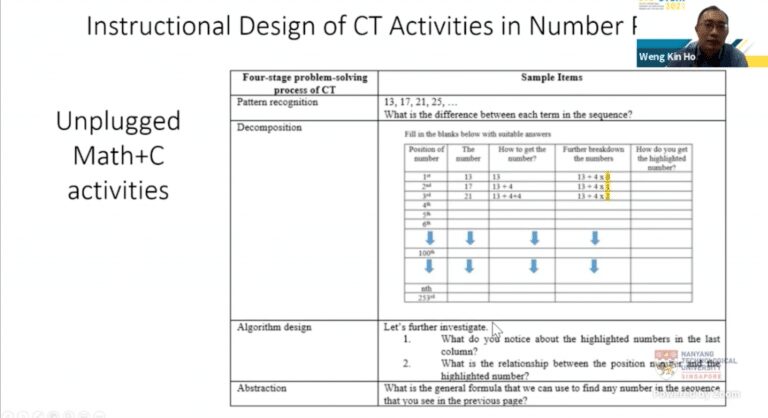
A/Prof Ho Weng Kin demonstrated how CT may be effortlessly integrated into the math curriculum in a keynote session at the CTE-STEM conference, and offered a compelling success story from Singapore. A/Prof Ho, a mathematician by training, chose the most important but otherwise mundane topic: numerals. Because the formal concept of rationality can be abstract and only accessible to students who are more mathematically advanced, A/Prof Ho decided to allow students to develop an intuition for irrational numbers through inductive discovery, using Microsoft Excel to iteratively “grow” their own irrational number. The irony in the kids’ responses may have added to his success: “If only every math class could be this exciting.”
Forum for Teachers
Apart from the keynote, Day 3 of the conference was a highlight for instructors searching for practical ideas to take back to their classrooms. Educators from all around the region attended the Teachers’ Forum, including those from India, Hong Kong, Indonesia, Thailand, Taiwan, and China, each giving their own perspective on CT in their own socio-cultural context. The impact of the internationally recognized Bebras Challenge and the Gerakan PANDAI, a domestic campaign to give CT professional development to 22,000 primary and junior high school teachers, was evaluated by Mulyanto and colleagues. Unplugged activities that do not require a computer (including calculators) were ingeniously used in rural schools where individual access to computers is a luxury (Chang & Guo). Palaparthi described how CT was implemented at charity schools in Andhra Pradesh, India, where kids played algorithmic games to determine a birthdate, inadvertently triggering a binary search.
A lesson study on incorporating CT in math lessons was conducted by Lee, Tang, and Pang from Singapore’s School of Science and Technology. They taught 51 Secondary students in this class. Two students of mixed to high ability were taught the fundamentals of quadratic functions as well as Python programming for seven hours.
There was clear evidence in the students’ work and written feedback that they practiced decomposition and, on occasion, drew relationships between the different qualities (pattern recognition) and directly related the coefficients of the equation to the graph property (abstraction). Despite the fact that not all groups were successful in supplying complete code, the students displayed a knowledge of the requirement for an algorithm and were able to relate components of algorithm creation to mathematical procedures such as completing the square.
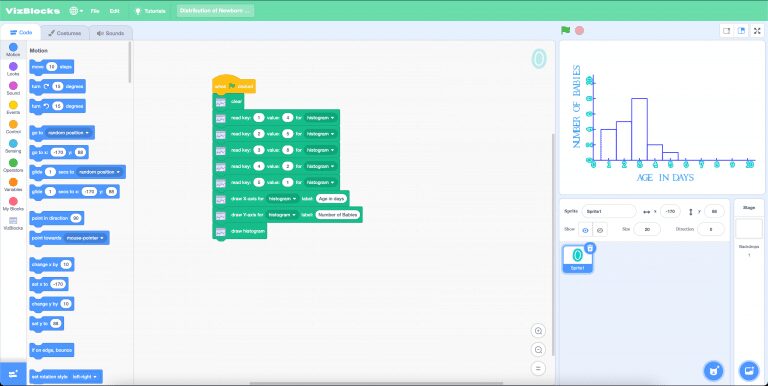
Vizblocks, a data visualization literacy tool built by an ambitious NUS undergraduate Travis Ching, is compatible with Scratch and will please math teachers. Vizblocks presently provides eight visualizations, which cover a wide range of graphical representations seen in the math curriculum. Students become increasingly aware of essential elements of such graphs as they get more actively involved in the creation process. Vizblocks also includes teaching materials such as instructional slides and worksheets for teachers who are worried about pedagogical support.
Conclusion
Take a step back and ask yourself, “Isn’t CT exactly what we’ve been attempting to achieve in problem-solving all along?” There are many parallels between CT and mathematical thinking, but the differentiating aspect of computability provides a genuine learning context. If you’re a math teacher, we hope you’ve left feeling a little more inspired and convinced of the value of CT.









